The Kalam Cosmological Argument is an argument for the existence of God that is broken up into two stages. The first stage of the argument tries to show that the universe has a cause and can be formulated as follows:
Whatever begins to exist has a cause.
The universe began to exist.
Therefore, the universe has a cause.
The second stage of the argument then attempts to derive various of the divine attributes from the cause of the universe, thus implying that the cause in question is plausibly taken to be God as classically conceived. Famously, St. Thomas Aquinas rejected this argument, while his contemporary St. Bonaventure endorsed it. Perhaps the most prominent defender of the Kalam today is the philosopher William Lane Craig. Craig typically offers two philosophical arguments in support of the second premise of the first stage of the argument (see the syllogism above). In this post, I want to examine Craig's first philosophical argument.
An actual infinite cannot exist
Craig’s first argument is that an actually infinite (as opposed to a merely potentially infinite) multitude of things cannot exist and that, if the universe did not begin to exist, then the past would consist of an actually infinite number of events. Thus, the past must not be infinite, and the universe must have begun to exist. The basic argument can be formulated as follows:
An actual infinite cannot exist.
An infinite temporal regress of events is an actual infinite.
Therefore, an infinite temporal regress of events cannot exist.
To start out, we must get clear on the distinction between an actual infinite and a potential infinite. Craig explains the distinction as follows:
There is a difference between a potential infinite and an actual infinite. A potential infinite is a collection that is increasing toward infinity as a limit but never gets there. Such a collection is really indefinite, not infinite. For example, any finite distance can be subdivided into potentially infinitely many parts. You can just keep on dividing parts in half forever, but you will never arrive at an actual “infinitieth” division or come up with an actually infinite number of parts. By contrast, an actual infinite is a collection in which the number of members is really infinite. The collection is not growing toward infinity; it is infinite, it is “complete.” This sort of infinity is used in set theory to designate sets that have an infinite number of members, such as {1, 2, 3 . . .}. Now the argument is, not that a potentially infinite number of things cannot exist, but that an actually infinite number of things cannot exist (Time and Eternity, pg. 221).
This is a very important distinction to keep in mind as we evaluate Craig’s arguments.
Another important point is to note what is meant by “event” in the second premise when we speak of an infinite temporal regress of events. Craig explains what he has in mind as follows:
By an “event,” one means any change. Since any change takes time, there are no instantaneous events so defined. Neither could there be an infinitely slow event, since such an “event” would, in reality, be a changeless state. Therefore, any event will have a finite, nonzero duration. In order that all the events comprised by the temporal regress of past events be of equal duration, one arbitrarily stipulates some event as our standard and, taking as our point of departure the present standard event, we consider any series of such standard events ordered according to the relation earlier than. The question is whether this series of events comprises an actually infinite number of events or not. If not, then since the universe cannot ever have existed in an absolutely quiescent state, the universe must have had a beginning. It is therefore not relevant whether the temporal series had a beginning point (a first temporal instant). The question is whether there was in the past an event occupying a nonzero, finite temporal interval which was absolutely first, that is, not preceded by any equal interval (The Blackwell Companion to Natural Theology, pp. 106).
With these explications in mind, we proceed to an evaluation of the argument.
Defense of first premise
In defense of the first premise, Craig tries to show that the existence of an actual infinite would entail various metaphysical absurdities. Insofar as these absurdities seem to be impossible, they provide evidence that an actual infinite cannot exist.
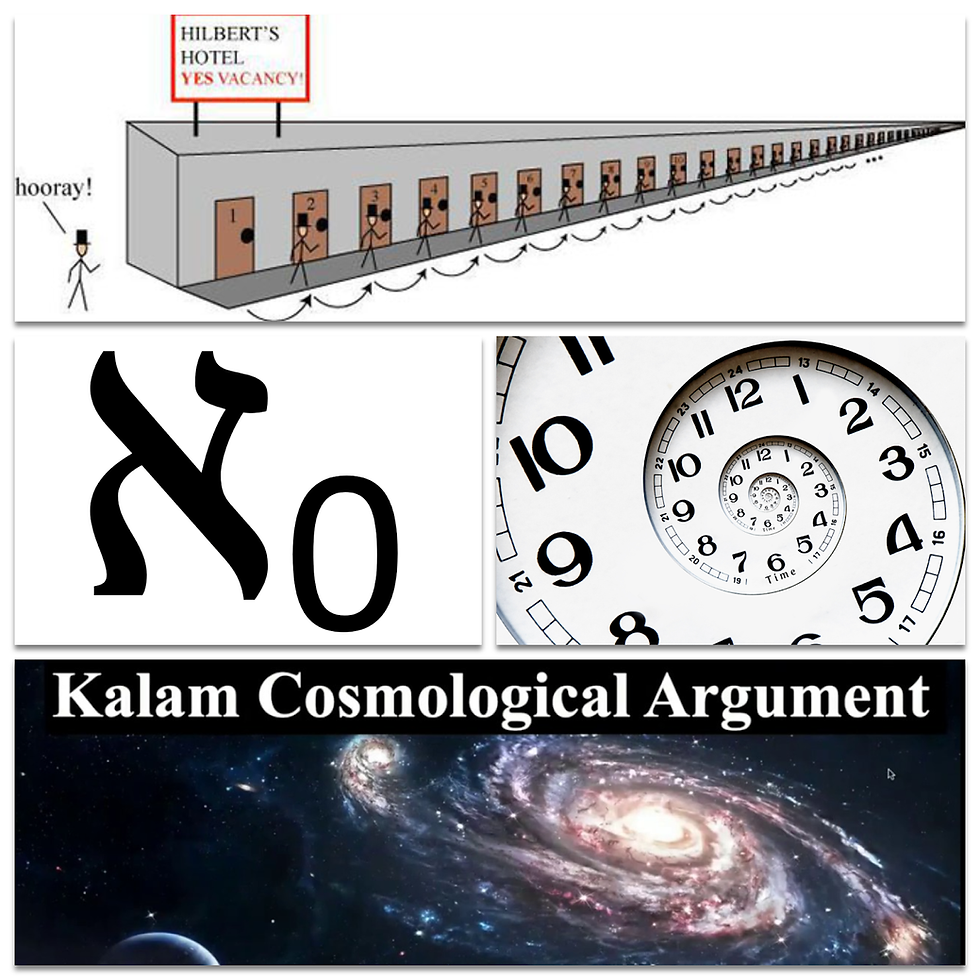
Hilbert’s Hotel
A favorite thought experiment of Craig’s that he uses to illustrate the absurdities of an actual infinite is Hilbert’s Hotel, which was originally developed by the twentieth-century German mathematician David Hilbert.
Before discussing Hilbert’s Hotel, some mathematical preliminaries are needed. Hilbert’s Hotel is mathematically cashed out in terms of set theory. We can understand a set S, for our purposes, as a collection of distinct, definite, and discrete objects called elements. The cardinality of a set S is the number of elements in the set and is denoted by |S|. A set can be either finite (containing a finite number of elements) or infinite (containing an infinite number of elements). An example of an infinite set directly relevant to Hilbert’s Hotel is the set of natural numbers, denoted by ℕ = {1, 2, 3, …}. The cardinality of ℕ is a transfinite number. Transfinite numbers have the property of being larger than any finite number. Such numbers are actual infinities. The smallest transfinite number is denoted by ℵ0 (pronounced “aleph-null”) and is equal to the cardinality of ℕ. Thus, we can write |ℕ| = ℵ0.
The sizes (i.e., cardinalities) of two sets can be compared in two different ways. The first and most obvious way is to simply count the elements in each set and compare the resulting numbers. However, while this method works for finite sets, it does not work for infinite sets, since we cannot count an infinite number of elements. Fortunately, the German mathematician Georg Cantor, the father of infinite set theory, came up with another method of comparing the sizes of two sets that happily does work for both finite and infinite sets. Consider two sets, X and Y. We can determine whether |X| = |Y| by determining whether the elements in X can be put into a one-one correspondence with the elements in Y. A one-one correspondence means that each element in X is paired with a unique element in Y and every element in Y is paired with a unique element in X. If a one-one correspondence can be given, then the sets have the same cardinality; otherwise, the sets do not have the same cardinality. For example, consider the sets X = {1, 3, 5} and Y = {2, 4, 6}. A one-one correspondence for these sets can be given as follows:
X | Y |
1 | 2 |
3 | 4 |
5 | 6 |
Figure 1: a one-one correspondence between X and Y
Thus, we may conclude that |X| = |Y|. When applying this method to infinite sets, counter-intuitive results can follow. For instance, consider the set of all even natural numbers E = {2, 4, 6, …}. Intuitively, it seems that |ℕ| > |E|. In fact, however, it can be shown that |ℕ| = |E| by way of the following one-one correspondence:
ℕ | E |
1 | 2 |
2 | 4 |
3 | 6 |
... (etc.) | ... (etc.) |
Figure 2: a one-one correspondence between ℕ and E
Thus, we may conclude that |ℕ| = |E|. Similar reasoning allows us to conclude that |ℕ| = |O|, where O is the set of all odd natural numbers, {1, 3, 5, …}. It is precisely counter-intuitive results such as these that Hilbert’s Hotel makes use of. An example of an infinite set that cannot be put into a one-one correspondence with ℕ is the set of real numbers, ℝ. Cantor, in fact, famously proved that |ℝ| > |ℕ|, thus proving the remarkable fact that some infinities are larger than others.
Finally, there are two set-theoretic operations that are relevant to Hilbert’s Hotel: set union and set difference. The set union of two sets X and Y, denoted by X ∪ Y, is equal to the set of all elements that are in either X or Y. In effect, the union of two sets combines the elements in both sets together into one set. For example, consider the sets X = {1, 3, 5} and Y = {2, 4, 6}. Then, X ∪ Y = {1, 2, 3, 4, 5, 6}. The set difference of two sets X and Y, denoted by X \ Y (the ordering of X and Y, unlike with respect to set union, is crucial here), is equal to the set of all elements in X that are not in Y. For example, consider the sets X = {1, 2, 3, 4, 5, 6} and Y = {1, 3, 5}. Then, X \ Y = {2, 4, 6}.
With these mathematical preliminaries in place, we can at last discuss Hilbert’s Hotel. Any additional mathematical concepts will be explicated as needed in the course of the discussion. The Hilbert’s Hotel thought experiment has us imagine a hotel with an actually infinite (ℵ0) number of rooms, all of which are occupied with guests. The full hotel, therefore, can be modeled as the set of all natural numbers, ℕ, with each natural number corresponding to a guest. There are four different scenarios that Craig considers:
Scenario 1: Accommodating a new guest
In this scenario, all of the rooms of the hotel are full. A new guest then arrives and wants a room. This can be accommodated by having the guest in room #1 move into room #2, the guest in room #2 move into room #3, and so on to infinity. The result will be that room #1 is vacant and the new guest can check into it. So, a new guest can check in even though all the rooms were full and not a single guest checked out of the hotel. Furthermore, according to infinite set theory, the number of guests in the hotel after the new guest checks in is the same as the number of guests before the new guest checks in, namely, ℵ0. The accommodation of the new guest mathematically corresponds to constructing a one-one correspondence between the sets ℕ and {2, 3, 4, …} (pair 1 with 2, 2 with 3, 3 with 4, etc.) and the set-theoretic operation of {2, 3, 4, …} ∪ {g}, where g is an element not in ℕ corresponding to the new guest. And |{2, 3, 4, …} ∪ {g}| = ℵ0.
Scenario 2: Accommodating an infinity of new guests
Similar to the first scenario, all of the rooms of the hotel are full. This time, however, instead of a single new guest wanting to check in, there are an infinity of new guests wanting to check in. This can be accommodated by having each guest in the hotel move to the room number that is twice their current room number. So, the guest in room #1 moves to room #2, the guest in room #2 moves to room #4, the guest in room #3 moves to room #6, and so on to infinity. The result will be that all of the infinitely many odd numbered rooms are empty and so the infinitely many new guests can all check in to the odd numbered rooms. And again, the number of guests in the hotel, according to infinite set theory, remains the same: ℵ0. The accommodation of an infinity of new guests mathematically corresponds to constructing a one-one correspondence between ℕ and E (as was shown in Figure 2 above) and the set-theoretic operation of {2, 4, 6, …} ∪ G, where G is an infinity of new guests and hence |G| = ℵ0. And |{2, 4, 6, …} ∪ G | = ℵ0.
Scenario 3: Odd numbered guests check out
In this scenario, the hotel is full, and all of the odd numbered guests check out. According to infinite set theory, the number of guests in the hotel is the same before the guests check out as after the guests check out, namely, ℵ0. Furthermore, the remaining guests can be shifted around so that all of the rooms are full again without any additional guests checking into the hotel. The procedure would simply involve moving each of the remaining even numbered guests into the room number that is half their current room number. So, the guest in room #2 moves to room #1, the guest in room #4 moves to room #2, and so on to infinity. The checking out of all of the odd numbered guests mathematically corresponds to the set-theoretic operation of ℕ \ O = E, where |ℕ| = ℵ0, |O| = ℵ0, and |E| = ℵ0. Hence, the number of guests in the hotel is the same before the guests check out as after the guests check out. The subsequent re-filling of the hotel mathematically corresponds to constructing a one-one correspondence between E and ℕ.
Scenario 4: Guests numbered greater than three check out
In the final scenario, the hotel is full, and all of the guests in rooms #4 and up check out of the hotel. The result is that there are now only three guests in the hotel. But, according to infinite set theory, the number of guests that checked out in this scenario is the same as the number that checked out in the previous scenario (ℵ0), even though the number of guests remaining differs in quantity in each case (ℵ0 and 3, respectively). Thus, we have subtracted identical quantities from identical quantities (ℵ0 – ℵ0) and have arrived at contradictory results. The checking out of all of the guests in rooms #4 and up mathematically corresponds to the set-theoretic operation of ℕ \ {4, 5, 6, …} = {1, 2, 3}, where |ℕ| = ℵ0, |{4, 5, 6, …}| = ℵ0, and |{1, 2, 3}| = 3.
To conclude, Craig writes:
Can anyone believe that such a hotel could exist in reality? Hilbert’s Hotel is absurd. But if an actual infinite were metaphysically possible, then such a hotel would be metaphysically possible. It follows that the real existence of an actual infinite is not metaphysically possible (ibid., pp. 109-110).
Critique of first premise
Jimmy Akin, a prominent Catholic apologist, offers a number of interesting critiques of Craig’s philosophical arguments for the finitude of the past. With respect to the first premise of the argument presently under consideration, Akin points out that the various scenarios with Hilbert’s Hotel certainly seem to be counter-intuitive and maybe even absurd in an informal sense. However, this does not seem to be enough to prove that such scenarios are truly metaphysically impossible. Furthermore, what sounds absurd is somewhat person-relative. What may seem absurd to one person may not seem absurd to another. So, whether or not something seems absurd or counter-intuitive does not seem to be a reliable guide to what is or is not metaphysically possible (“Checking out of Hilbert’s Hotel”).
In response to this, it is certainly true that what sounds absurd is going to tend to be somewhat person-relative and subjective. Craig himself seems to admit as much when he writes as follows:
Arguments for metaphysical possibility or impossibility typically rely upon intuitions and conceivability arguments, which are obviously much less certain guides than strict logical consistency or inconsistency…[A]rguments for the metaphysical impossibility of some state of affairs will be much more subjective than arguments concerning strict logical impossibility (Blackwell Companion, pp. 106).
Given this, the first two scenarios involving Hilbert’s Hotel do seem to be inconclusive with respect to strictly demonstrating the metaphysical impossibility of an actual infinite. However, the last two scenarios seem to jointly imply a genuine logical contradiction. For in these scenarios, we are subtracting identical quantities from identical quantities and getting different results. Since such a logically impossible state of affairs would be possible if an actual infinite could exist, it must be the case that an actual infinite really cannot exist.
In response to this, Akin offers the following reply (where he uses an example involving an actually infinite number of apples rather than hotel rooms):
Suppose that you go through my apple collection and pull out all the odd numbered apples (let’s suppose that I’ve conveniently numbered them so I can always find the apple I want). How many are left in the collection? Pulling out every other apple from an unlimited number of apples would still leave an unlimited number there, so my collection is still of unlimited size. And I can re-number the apples I have left if I want.
Finally, suppose that you pull all of the apples out of my collection except the first three. How many do I have left? Three. “Why is the number different than when I pulled out the odd numbered ones?” you might ask. “Because of which ones you pulled out,” I reply. “The first time, you pulled out every other apple, but the second time you pulled out all of the apples but the first three. Of course, you’re going to get a different number left over at the end.” (“Checking out of Hilbert’s Hotel”)
So, according to Akin, the fact that there are different results that are arrived at by subtracting out the odd numbered guests (or apples) versus subtracting out all the guests (or apples) greater than three does not amount to a contradiction because we are using different subtraction procedures in each case. The different results are thus explained by the fact that we are using different procedures. Thus, there is no contradiction. Craig anticipates this objection and responds as follows to a version of it given by David Yandell:
It is, of course, true that every time one subtracts all the even numbers from all the natural numbers, one gets all the odd numbers, which are infinite in quantity. But that is not where the contradiction is alleged to lie. Rather the contradiction lies in the fact that one can subtract equal quantities from equal quantities and arrive at different answers. For example, if we subtract all the even numbers from all the natural numbers, we get an infinity of numbers, and if we subtract all the numbers greater than three from all the natural numbers, we get only four numbers. Yet in both cases we subtracted the identical number of numbers from the identical number of numbers and yet did not arrive at an identical result. In fact, one can subtract equal quantities from equal quantities and get any quantity between zero and infinity as the remainder. For this reason, subtraction and division of infinite quantities are simply prohibited in transfinite arithmetic—a mere stipulation which has no force in the nonmathematical realm (Blackwell Companion, pp. 112).
What should we make of Akin’s objection and Craig’s reply to it? Here, it will be helpful to additionally consider Louis J. Swingrover’s objections against Craig’s appeal to Hilbert’s Hotel (“Difficulties with William Lane Craig’s Arguments for Finitism”). Swingrover’s argument can be seen as a mathematical fleshing out of Akin’s objection. Swingrover argues that Craig’s attempt to use Hilbert’s Hotel to derive a logical contradiction fails because it equivocates on performing the arithmetical operation of subtraction on the cardinal numbers of two sets and performing the set-theoretic operation of set difference (alternatively, relative complement) on the elements of two sets. The contradiction that Craig seeks to derive can be formulated as follows:
Case 1:
Start with a full Hilbert’s Hotel. Represent the guests in the hotel by the set of natural numbers, ℕ = {1, 2, 3, …}. Note that |ℕ| = ℵ0.
Have all of the odd numbered guests check out of the hotel. Represent the odd numbered guests by the set O = {1, 3, 5, …}. Note that |O| = ℵ0. Represent the odd numbered guests checking out by performing the set difference operation ℕ \ O = E = {2, 4, 6, …}. Note that |E| = ℵ0.
Since we started with ℵ0 elements, removed ℵ0 elements, and were left with ℵ0 elements, we can represent the quantity of guests that we started with, that checked out, and that remain by the equation E1: ℵ0 – ℵ0 = ℵ0.
Case 2:
Start with a full Hilbert’s Hotel. Represent the guests in the hotel by the set of natural numbers, ℕ = {1, 2, 3, …}. Note that |ℕ| = ℵ0.
Have all of the guests numbered greater than three check out of the hotel. Represent these guests by the set S = {4, 5, 6, …}. Note that |S| = ℵ0. Represent the guests numbered greater than three checking out by performing the set difference operation ℕ \ S = T = {1, 2, 3}. Note that |T| = 3.
Since we started with ℵ0 elements, removed ℵ0 elements, and were left with 3 elements, we can represent the quantity of guests that we started with, that checked out, and that remain by the equation E2: ℵ0 – ℵ0 = 3.
Conclusion: From the equations E1 and E2, we can derive the conclusion that ℵ0 = 3, which is a contradiction. We are, as Craig says, subtracting identical quantities from identical quantities and getting different results.
The error in this reasoning, as Swingrover sees it, is that the set difference of two sets is not the same thing as the subtraction of the cardinalities of those sets. The operation being performed in the Hilbert’s Hotel scenarios is set difference, not subtraction. With respect to set difference, we are removing particular elements in a set. And with respect to the above two cases, we are importantly not removing identical elements from identical sets. Rather, we are removing different elements in each case. So, we are not performing identical operations and getting different results as Craig claims. Given this, it shouldn’t be much of a surprise that the resultant sets have different cardinalities. For we are not in the first place subtracting cardinalities; rather, we are removing elements from sets via the set difference operation. This seems to flesh out and make more mathematically precise Jimmy Akin’s earlier claim that the reason that the number of guests (or apples, as used in his example) differs in each case is because of which ones were removed. Thus, the move from step (2) to step (3) in each case is invalid. As Swingrover states, “[O]ne cannot infer the cardinality of the difference between the cardinalities of two sets from the cardinality of the relative complement [i.e., set difference] of one of the sets in the other” (ibid., pg. 12). In particular, it is not true that for two sets X and Y, |X \ Y| = |X| – |Y|.
Swingrover demonstrates this by way of constructing a counterexample as follows:
Let X = {1, 2, 3}.
Let Y = {2, 3, 4}.
Let Z = X \ Y = {1}.
|X| = 3
|Y| = 3
|Z| = 1
|X| – |Y| = 0
Assume for reductio that |X \ Y| = |X| – |Y|.
Then, |Z| = |X| – |Y| → 1 = 0. Contradiction.
Therefore, |X \ Y| ≠ |X| – |Y|.
Now, Craig’s reasoning relies on the equation expressed in (8). Since, therefore, this equation has just been shown to be false, it follows that Craig’s reasoning is invalid.
In response to this, it must be pointed out that while Swingrover is correct that in general the equation in question does not hold, he overlooks a special case in which it does hold, namely, when Y is a subset of X, which is denoted by Y ⊆ X. For two sets X and Y, Y ⊆ X if every element of Y is also an element of X. Thus, it is true to say that, for two sets X and Y, Y ⊆ X → |X \ Y| = |X| – |Y|. Now, in Craig’s argument, O ⊆ ℕ (Case 1) and S ⊆ ℕ (Case 2). Thus, equations and E1 and E2 seem to hold after all. Unfortunately for Craig, however, the statement that Y ⊆ X → |X \ Y| = |X| – |Y| is true only for finite sets, not infinite sets. Hence, equations and E1 and E2 do not hold, and the contradiction that Craig derives from them is thus moot. Therefore, it has not been shown that Hilbert’s Hotel entails a logical contradiction.
Moreover, it should be stated that transfinite arithmetic does not really “prohibit” inverse operations (such as subtraction and division) as Craig claims so much as it simply leaves them undefined. The reason that these operations are undefined is because, given how, for instance, the addition operation is defined, natural definitions of subtraction, as Craig correctly points out, lead to contradictions so that an operation like ℵ0 – ℵ0 would be indeterminate. Recall that ℵ0 is a cardinal number. It represents the cardinality of the set of natural numbers and any set that can be put into a one-one correspondence with the set of natural numbers. Addition on cardinal numbers (including transfinite cardinal numbers) is defined as the cardinality of the union of two disjoint sets having the respective cardinalities that are being added together. Two sets are disjoint if they have no elements in common. Thus, consider the following operation which adds ℵ0 to itself: ℵ0 + ℵ0. To compute this, we can simply find two sets that are disjoint such that each of their cardinalities is equal to ℵ0, compute the union of those two sets, and then compute the cardinality of that union. The easy choice is to select the sets E and O, where E is the set of even natural numbers and O is the set of odd natural numbers. Clearly, |E| = ℵ0 and |O| = ℵ0. Furthermore, E and O are clearly disjoint. Thus, we can compute ℵ0 + ℵ0 as follows: ℵ0 + ℵ0 = |E ∪ O| = ℵ0. Such a notion of addition is entirely logically consistent both for finite and infinite cardinals. Furthermore, it is sensible and useful. For instance, for two finite cardinals, C1 and C2, C1 + C2 behaves just like standard addition on natural numbers.
How, then, might we try to define subtraction for cardinal numbers? A natural definition that suggests itself is that subtraction on cardinal numbers is defined as the set difference of two disjoint sets having the respective cardinalities that are being subtracted. The trouble with this is that it isn’t a very useful definition of subtraction because it would always result in the following, for two cardinal numbers (whether finite or infinite) C1 and C2: C1 – C2 = C1. Clearly, this is not at all how standard subtraction on natural numbers behaves. A seemingly more plausible alternative would be to simply define subtraction on cardinal numbers as the set difference of two sets having the respective cardinalities that are being subtracted. In this case, we have simply removed the disjoint requirement. Perhaps this definition will do the trick. Consider the following example:
X = {1, 3, 5}; |X| = 3.
Y = {2, 4, 6}; |Y| = 3.
Z = X ∪ Y = {1, 2, 3, 4, 5, 6}; |Z| = 6.
Z corresponds to the cardinal addition of 3 + 3 = 6. Now, let’s apply our subtraction operation:
6 – 3 = 3, which corresponds to either |Z \ X| = |Z| – |X| = |Y| or |Z \ Y| = |Z| – |Y| = |X|.
Notice, though, that we’ve already seen that these equations do not in general hold. They only hold when X ⊆ Z and Y ⊆ Z hold, respectively, and even then, only if X, Y, and Z are finite sets. Otherwise, in general, this subtraction procedure will yield contradictory results depending on the sets that are used, and this is true even for finite sets. It would seem, then, that there really isn’t a natural and useful definition of subtraction on cardinal numbers, which is why transfinite arithmetic does not define subtraction.
In response to all of this, there is possibly one remaining strategy that Craig could adopt in order to salvage his argument. He could forgo trying to formally define and perform subtraction on cardinal numbers and instead simply insist on focusing on set difference and determining the number of elements we started with, the number of elements we removed, and the number of elements that remain. So, consider again the two Hilbert’s Hotel operations: (1) Odd numbered guests check out, and (2) Guests numbered greater than three check out. Whether we are performing the mathematical operation of arithmetic subtraction or the mathematical operation of set difference, the point is that in both Hilbert’s Hotel cases, we are starting with a set of size ℵ0 and are removing ℵ0 elements and are getting two different numbers of elements, ℵ0 and 3, respectively, remaining, and this is a contradiction.
But there are three problems with this response: (1) The response treats ℵ0 as if it were a regular natural number, a fixed and entirely determinate quantity. But this is not the case. This can be seen by taking note of the fact that, for instance, ℵ0 + 1 = ℵ0. (2) The response treats cardinal arithmetic as if it were the same as standard arithmetic on the natural numbers. Leaving aside the aforementioned problems with trying to define subtraction on cardinal numbers, addition on cardinal numbers is not the same operation as addition on natural numbers. This can be seen from the set-theoretic definition of addition on cardinal numbers. Furthermore, while addition on cardinal numbers behaves like addition on natural numbers for finite cardinal numbers, this is not the case for transfinite cardinals. (3) The response, if sound, would prove too much. For it would prove that the set difference operation itself entails contradictions, which would mean that set difference would not be a valid operation. Hence, the two Hilbert’s Hotel scenarios could not even get off the ground since they explicitly rely on performing the set difference operation. Moreover, when we ignore cardinalities, the set difference operation is clearly perfectly sensible and well-defined. Even with infinite sets, set difference always yields completely consistent and determinate results. It is only when we try to read cardinalities off of the set difference operation that we land in contradictions. The takeaway from this is not that there is anything suspect with set difference but rather that one simply cannot read off cardinalities from set difference.
For all of these reasons, therefore, it seems that Craig’s attempt to derive a logical contradiction from Hilbert’s Hotel fails. Does this mean that Hilbert’s Hotel does not after all show that an actual infinite cannot exist? Not necessarily. For, as aforementioned, it may be that Hilbert’s Hotel, while strictly logically possible, is nevertheless metaphysically impossible. Hilbert’s Hotel is certainly extremely counter-intuitive. Is this enough, however, to conclude that it is metaphysically impossible? I think that answering this question will ultimately come down to a battle of intuitions. Some may be persuaded that Hilbert’s Hotel is metaphysically impossible, whilst others will not be. Apologetically, therefore, Hilbert’s Hotel does, I think, have value. But it is a relative value, not an absolute value. Taken as a suggestive thought experiment, Hilbert’s Hotel potentially has genuine dialectical force. Taken as a strict demonstration, however, I think that Hilbert’s Hotel is inconclusive with respect to establishing the truth of the first premise of the argument presently under consideration, namely that An actual infinite cannot exist.
The philosophers Alex Malpass and Wes Morriston critique the first premise by arguing that if there is something suspect with an infinite past, then there is something equally suspect with an infinite future. But, since there does not appear to be anything suspect with an infinite future, it follows that neither is there anything suspect with an infinite past. And since an infinite past would constitute an actual infinite, the existence of an actual infinite turns out to be possible after all. The upshot is that the first premise of Craig’s argument is false. In defense of this contention, Malpass and Morriston construct an argument parallel to Craig’s argument. But instead of arguing against the possibility of a beginningless past, the parallel argument is made against the possibility of an endless future. The argument is presented as follows:
An actual infinite cannot exist.
An infinite temporal progress of events is an actual infinite.
Therefore, an infinite temporal progress of events cannot exist (“Endless and Infinite”, pp. 831).
Morriston defended a similar objection in a prior article as well (“Beginningless Past, Endless Future, and the Actual Infinite”). Craig’s response is to argue that an infinite temporal regress of events would be an actual infinite, whereas an infinite temporal progress of events would merely be a potential infinite. Thus, there is a crucial asymmetry between past and future (“Taking Tense Seriously in Differentiating Past and Future: A Response to Wes Morriston”). Craig writes,
There are two ways in which a temporal series of…events which has a beginning might be endless: (i) it might be actually infinite, that is to say, composed of an actually infinite number of events; (ii) it might be potentially infinite, that is to say, composed of a finite but ever increasing number of events with infinity as a limit. The second answer entails an A-theory of time according to which temporal becoming is an objective feature of reality, whereas the first answer is naturally associated with a B-theory according to which all events in time are on an ontological par (ibid.).
This is where the nature of time becomes crucial in evaluating Craig’s philosophical arguments and the objections raised against them by his critics. If the A-Theory of time—particularly presentism—is correct, then there is a crucial asymmetry between past and future. A beginningless past would constitute an actually infinite number of events that have transpired, whereas an endless future would constitute a potentially infinite number of events that will transpire. It simply will never be the case that an actually infinite number of events will occur on the supposition of a finite past but an endless future. Craig elaborates on these ideas as follows:
Accordingly, one might be tempted to say that in an endless future there will be an actually infinite number of events, just as in a beginningless past there have been an actually infinite number of events. But in a sense that assertion is false; for there never will be an actually infinite number of events since it is impossible to count to infinity. The only sense in which there will be an infinite number of events is that the series of events will go toward infinity as a limit. But that is the concept of a potential infinite, not an actual infinite. Here the objectivity of temporal becoming makes itself felt. For as a result of the arrow of time, the series of events later than any arbitrarily selected past event is properly to be regarded as potentially infinite, that is to say, finite but indefinitely increasing toward infinity as a limit. The situation, significantly, is not symmetrical: as we have seen, the series of events earlier than any arbitrarily selected future event cannot properly be regarded as potentially infinite…Ironically, then, it turns out that the series of future events cannot be actually infinite regardless of the infinity of the past or the metaphysical possibility of an actual infinite, for it is the objectivity of temporal becoming that makes the future potentially infinite only (Blackwell Companion, pp. 116).
Given the asymmetry between past and future, an endless future does not amount to an actual infinite like a beginningless past does. The objections of Malpass and Morriston, therefore, do not succeed.
Defense of second premise
But what of the second premise? Craig seems to take it to be rather obviously true. He writes,
The second premise states than an infinite temporal regress of events is an actual infinite. The point seems obvious enough, for if there has been a sequence composed of an infinite number of events stretching back into the past, then the set of all events in the series would be an actually infinite set (Blackwell Companion, pp. 115).
Critique of second premise
While the second premise may seem obvious to Craig, it has not seemed so to others. The (Thomist) philosopher Edward Feser, for instance, criticizes the second premise on the grounds that, given a presentist ontology of time (which, as aforementioned, Craig’s formulation of the KCA presupposes), an infinite temporal regress of events is not an actual infinite in the sense that Craig’s argument relies on. This is because on presentism, the present alone actually exists. The past no longer exists, and the future does not yet exist (“A difficulty for Craig’s kalām cosmological argument?”). The first premise of Craig’s argument is that an actual infinite cannot exist (that is, exist in the “real world”). And the Hilbert’s Hotel scenarios that Craig offers in defense of the first premise rely on there being an actually infinite multitude of things existing in the real world here and now. But this condition does not hold with respect to the series of past events, since past events (again, given presentism) do not actually exist. So, the absurdities of examples like Hilbert’s Hotel simply do not arise with respect to the series of past events, even supposing an infinite past.
Feser notes that Craig’s argument seems to confuse the following two claims regarding an actually infinite past:
The number of moments that have actually existed is infinite.
The number of moments that actually exist is infinite.
Feser observes that a beginningless universe (on presentism) would entail the first claim but not the second claim. And it is the second claim that is needed in order for an infinite past to be relevantly similar to examples like Hilbert’s Hotel (“Yeah, but is it actually actually infinite?”).
Craig anticipates this objection and replies to it as follows:
The question…is whether events’ temporal distribution over the past on a presentist ontology precludes our saying that the number of events in a beginningless series of events is actually infinite.
Now we may take it as a datum that the presentist can accurately count things that have existed but no longer exist. He knows, for example, how many US presidents there have been up through the present incumbent, what day of the month it is, how many shots Oswald squeezed off, and so forth. He knows how old his children are and can reckon how many billion years have elapsed since the Big Bang, if there was such an event. The nonexistence of such things or events is no hinderance to their being enumerated. Indeed, any obstacle here is merely epistemic, for aside from considerations of vagueness there must be a certain number of such things. So in a beginningless series of past events of equal duration, the number of past events must be infinite, for it is larger than any natural number. But then the…past events…in an infinite temporal regress of events…constitute an actual infinite…
Because the series of past events is an actual infinite, all the absurdities attending the existence of an actual infinite apply to it. For example, if the series of past events is actually infinite, then the number of events that have occurred up to the present is no greater than the number that have occurred at any point in the past. Or again, if we number the events beginning in the present, then there have occurred as many odd-numbered events as events. If we mentally take away all the odd-numbered events, there are still an infinite number of events left over; but if we take away all the events greater than three, there are only four events left, even though in both cases we took away the same number of events (Blackwell Companion, pp. 115- 116).
But Craig’s response seems unpersuasive. For the fundamental point remains: past events, on presentism, do not exist. Yes, we can, nevertheless, enumerate them, but we can also enumerate the natural numbers. And Craig grants the logical consistency and mathematical legitimacy of the actual infinite with respect to set theory in particular and to mathematics in general. The reason that the actual infinite is legitimate in mathematics, according to Craig, is that mathematical objects don’t actually exist in the “real world.” But past events no more exist in the real world than mathematical objects do. Hence, if there is no issue with the set of natural numbers being actually infinite, then it seems that there is no issue with the series of past events being actually infinite. The fact that we can, as Craig says, “mentally take away” past events is no more relevant to establishing the absurdity of an actually infinite past than being able to mentally take away numbers is relevant to establishing the absurdity of an actual infinite set of numbers. Hilbert’s Hotel, which relies on an actual infinite existing in the real world all at once thus seems to be irrelevant to the metaphysical possibility of an infinite past. As Feser writes,
A beginningless series of events will be problematic…only if it is more like the collection of rooms in Hilbert’s hotel than it is like the series of natural numbers.
[T]he trouble with Craig’s position is that past things (whether events, years, or whatever) do not exist, at least not given presentism. So they are not relevantly like the concrete objects which all exist together in the Hilbert’s hotel example. Craig’s reply here thus seems to me to ignore the objection from presentism rather than answering it (“Yeah, but is it actually actually infinite?”).
Furthermore, as Jimmy Akin points out, Hilbert’s Hotel is disanalogous to an infinite past for another reason as well: the past cannot be changed, not even by God. Recall that omnipotence (the attribute in virtue of which God is all-powerful) does not entail that God can bring about logical impossibilities such as square circles and married bachelors. Especially on a presentist ontology of time, it seems that changing the past amounts to a logical contradiction, and, therefore, even God cannot change the past. St. Thomas Aquinas argued for this conclusion as follows:
[T]here does not fall under the scope of God’s omnipotence anything that implies a contradiction. Now that the past should not have been implies a contradiction. For as it implies a contradiction to say that Socrates is sitting, and is not sitting, so does it to say that he sat, and did not sit. But to say that he did sit is to say that it happened in the past. To say that he did not sit, is to say that it did not happen. Whence, that the past should not have been, does not come under the scope of divine power (Summa Theologica, Pt. I, Q. 25, Art. 4).
Given this, Akin explains the disanalogy between Hilbert’s Hotel and an infinite past:
God cannot…cut up, rearrange, and reshuffle the moments of history the way Hilbert’s hotelier moved guests around…
God cannot…create or delete additional moments in time beyond those he has already created…
That means that the kind of puzzles that arise with Hilbert’s Hotel simply do not arise with time…
An actually infinite history for the universe thus would go in the same category as an actually infinite number of mathematical truths. It does not give rise to Hilbert paradoxes…
Therefore, even if one grants that Hilbert’s Hotel involves a logical contradiction and could not be realized by an omnipotent God, it does not matter…[A]n infinite amount of time (past or future) does not generate the problems of Hilbert’s Hotel and so is not in the same category (“Checking out of Hilbert’s Hotel”).
Craig himself emphasizes that Hilbert’s Hotel shows that an actual infinite cannot exist in reality because of the absurd results that could follow from it. This is in contrast to a purely mathematical actual infinite. As aforementioned, Craig affirms that the actual infinite, so long as it is constrained to the mathematical world, poses no issues. This is because (at least seeming) absurdities such as subtracting infinite quantities from infinite quantities and getting different results do not arise in mathematics since inverse operations (e.g., subtraction and division) are "prohibited" in transfinite arithmetic. But this prohibition is a mere mathematical stipulation that “has no force in the nonmathematical realm” (Blackwell Companion pp. 112). In reality, there is nothing in principle preventing the guests of Hilbert’s Hotel from checking out. In Craig’s own words,
Now infinite set theory is strictly logically consistent, granted its axioms and rules, but that does nothing to prove that such a system can exist in the real world. This fact is especially evident when it comes to mathematical operations such as subtraction and division, which transfinite arithmetic must prohibit in order to maintain logical consistency. While we can slap the hand of the mathematician who attempts such operations with infinite numbers, we cannot in reality prevent people from checking out of a Hilbert’s Hotel with all the attendant absurdities (Time and Eternity, pg. 224).
But an infinite past is not like Hilbert’s Hotel in that there is something in principle preventing inverse operations from being applied to the series of past events: namely, the fact that it is metaphysically impossible to change the past. So, it seems that even if Hilbert’s Hotel is metaphysically impossible, this fact alone does not demonstrate that an actually infinite past is impossible, given the peculiar nature of the past on a presentist ontology.
This point may even cast doubt on the truth of the first premise of Craig’s argument, namely that An actual infinite cannot exist. For if a particular actual infinite existed such that it was metaphysically impossible to perform any kind of manipulations on it (such as subtraction), then the absurdities of Hilbert’s Hotel could not in principle arise with such an actual infinite. This point has been made, for instance, by Graham Oppy (Philosophical Perspectives on Infinity, pg. 52-53; Arguing About Gods, pg. 140). So, it may be that Hilbert’s Hotel at best only rules out certain kinds of actual infinities. But if the actual infinite in question involves an actually infinite collection of concrete objects (which is what the first premise seems to have in mind), then it is hard to see how manipulations of such objects would be metaphysically impossible. So, the first premise would seem to retain whatever plausibility it previously had. In any case, it seems that Hilbert’s Hotel is at least not analogous to an infinite past, and so an infinite past is not an actual infinite in the sense meant by the first premise. So, the second premise seems to be false.
While the first premise of Craig’s argument has some plausibility, the second premise is much more dubious. Craig’s first philosophical argument for the finitude of the past, therefore, seems inconclusive at best. With that being said, I do think that Craig's second philosophical argument for the beginning of the universe is sound. An evaluation of that argument, however, will have to wait for a future post.

Yorumlar